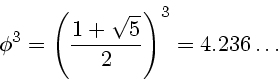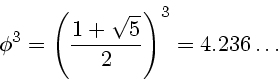11 The Subtle Mean

The quadratic PV number

$$\phi^3 = \left( {\frac{{1 + \sqrt 5 }}{2}} \right)^3 = 4.236 \ldots$$

appears frequently in diverse fields of knowledge. Its continued fraction expansion is purely periodic, that is,

$$\phi^3 = \left[ {\overline 4 } \right],$$

and is one of the most important Metallic Means, the positive solution of the equation

$$x^2 - 4x - 1 = 0.$$

It has been called the Subtle Mean by El Naschie [28] and, besides appearing in quasi periodic tiling and crystallography, it plays a significant role in the theory of Cantorian fractal-like micro-space-time

$$E^{(\infty )}$$

In fact, the mean value of the Hausdorff dimension of this space-time is precisely the Subtle Mean [29]

$$\left\langle {\dim E^{(\infty )} } \right\rangle = \phi^3 .$$

In addition, the Subtle Mean is involved in a fundamental way in various basic equations in knot theory, noncommutative geometry, and four manifold theory [30].