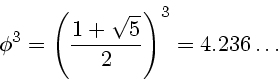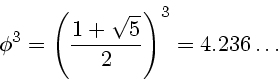Bibliography

1

The Metallic Means and Design, Nexus II: Architecture and Mathematics, Kim Williams Ed., 1998.

2

From the Golden Mean to Chaos, Nueva Libreria, 1998.

3

Pisot and Salem Numbers, Birkhauser, 1992.

4

Pisot-Cyclotomic Quasilattices and Their Symmetry Semigroups, in Quasicrystals and Discrete Geometry, Fields Institute Monographs, Jiri Patera Ed., American Mathematical Society, 1998.

5

Pisot-Cyclotomic Integers for Quasilattices, The Mathematics of Long-Range Aperiodic Order, R.V. Moody Ed., pp. 175-198, 1995.

6

Beta-Integers as Natural Counting Systems for Quasicrystals, J. Phys. A: Math. Gen., 1998, no 31, pp. 6449-6472.

7

``Triangulature" in Andrea Palladio, Nexus Network Journal, Architecture and Mathematics on line, Kim Williams Ed., 1999.

8

Localization Problem in One Dimension: Mapping and Escape, Phys. Rev. Lett., 1983, vol. 50, no 23, pp. 1870-1872.

9

Shape of Fractal Growth Patterns: Exactly Solvable Models and Stability Considerations, Phys. Rev. Lett., 1998, vol. 60, no. 24, pp. 2511-2514.

10

The Metallic Means Family and Multifractal Spectra, Nonlinear Analysis, 1999, vol. 36, pp. 721-745.

11

Electronic States on a Penrose Lattice, Phys. Rev. Lett., 1986, vol. 56., pp. 2740-2743.

12

Critical Wave Functions and a Cantor-Set Spectrum of a One-Dimensional Quasi-Crystal Model, Phys. Rev., B, 1987, vol 35, no. 3, pp. 1020-1033.

13

New Localization in a Quasi-Periodic System, Phys. Rev. Lett., 1989, vol. 62, no. 23.

14

Multifractal Wave Functions on a Fibonacci Lattice, Phys. Rev., B, 1989, vol. 40, no. 10, pp. 7413-7416.

15

Scaling Analysis of Quasi-Periodic Systems: Generalized Harper Model, Phys. Rev., B, 1989, vol. 40, no. 12, pp. 8225-8234,.

16

Energy Spectrum and the Quantum Hall Effect on the Square Lattice with Next-Nearest-Neighbor Hopping, Phys. Rev., B, 1990, vol. 42, no. 13, pp. 8282-8294.

17

Multifractal Method for Spectra and Wave Functions of Quasiperiodic Systems, ``Quasicrystals", T. Fujiwara and T. Ogawa Eds., Berlin: Springer-Verlag, 1990.

18

Electronic Spectra and Wave Function Properties of One-Dimensional Quasi-Periodic Systems: a Scaling Approach, Int. J. of Mod. Phys., B, 1992, vol. 6, no. 3 and no. 4, pp. 281-320.

19

Quantum Mechanics and the Possibility of a Cantorian Space-Time, Chaos, Solitons and Fractals, 1991, vol. 1, pp. 485-487.

20

Renormalization Approach to the Dimension of Diffusion in Cantorian Space, Appl. Math. Lett., 8, 1995, no. 1, pp. 59-63.

21

Average Symmetry Stability and Eergodicity of Multidimensional Cantor Sets, Nuovo Cimento, 109 B, 1994, no. 2.

22

Silver Mean Hausdorff Dimension and Cantor Sets, Chaos, Solitons and Fractals, 1994, no. 4, pp. 1862-1870.

23

Dimensions and Cantor Spectra, Chaos, Solitons and Fractals, 1994, no. 4, pp. 2121-2132.

24

Statistical Geometry of a Cantor Discretum and Semiconductors, Computers Math. Appl., 1995, vol. 29, no 12, pp. 103-110.

25

Quasiperiodic Dynamics for a Generalized Third-Order Fibonacci Series, Phys. Rev., B, 1998, vol. 38, no. 10, pp. 7091-7093.

26

Dynamical Maps, Cantor Spectra and Localization for Fibonacci and Related Quasiperiodic Lattices, Phys. Rev. Lett., 1998, vol. 60, no. 11, pp. 1081-1084.

27

Electronic Properties of the Tight-Binding Fibonacci Hamiltonian, J. Phys. A: Math. Gen., 1989, vol. 22, pp. 951-970.

28

COBE Satellite Measurement, Hyperspheres, Superstrings and the Dimension of Space Time, Chaos, Solitons and Fractals, 1998, vol. 9, no. 8, pp. 1445-1471.

29

Remarks to the PV Number

$$\phi^3 = \left( {\frac{{1 + \sqrt 5 }}{2}} \right)^3 = 4.236 \ldots$$

Chaos, Solitons and Fractals, 1999, vol. 10, no. 8, pp. 1335-1341.

30

The Golden Mean in Quantum Geometry, Knot Theory and Related Topics, Chaos, Solitons and Fractals, 1999, vol. 10, no. 8, pp. 1303-1307.

31

Number Theory in Science and Communication, with Applications in Cryptography, Physics, Digital Information, Computing and Self-Similarity, 3rd ed., Berlin: Springer, 1997.